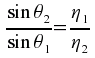
I have never been satisfied with the public explanations that one can find – on the web, for example – for the existence and location of rainbows. It's understandable that some of the technical detail is omitted, but it seems to me that too much is omitted. I therefore decided to do the calculations for myself. What you see here is the result.
Some of the basic facts are very well known. Most people know that white light is made up of many different colours, and that human eyes are able to see the light frequencies that correspond to the range from red to violet. Most of us also know that when a beam of light is refracted, as for example when it passes through a prism, the different colours are bent by slightly different angles, so that the result as the light comes out is not white light, but an continuum of colours.
A few extra facts can be established just by looking at a rainbow. You don't get a rainbow unless you have both sunlight and water droplets. (Well, OK, moonlight will sometimes work, but it's not bright enough to give the full effect.) The colours are arranged so that red is on the outside, and blue on the inside. The rainbow appears on the opposite side of the sky from the sun, so that you have the back of your head towards the sun when you're looking at the rainbow. (How many people remembered that, without having to go and check?) The rainbow is the arc of a circle, whose centre is on a line from the sun to your head. (So it moves when you move; you can never get to the end of a rainbow.) From the observer's viewpoint, the arc of the rainbow is displaced about 42 degrees from that centreline.
There is a secondary rainbow, at an angle of 50 degrees from the centreline, but it's not as easy to see because it's much less bright. The colours of the secondary rainbow are back to front, with the red on the inside. If you're really observant, you'll notice that the sky appears darker than average between the two rainbows, and brighter inside the primary rainbow.
All of that adds up to a partial explanation. Since we see the rainbow only where rain is falling, or where there's another source of water droplets such as a garden sprinkler, and since it's seen on the side away from the sun, the cause of the rainbow must be some sort of reflection from the water droplets. Straight reflection wouldn't do the trick, because that would not involve any sort of refraction. We need the refraction to separate the white light into its constituent colours. It seems likely, then, that we have a more complicated reflection, where the light enters the raindrop, bounces off the inside, and then emerges on a path towards your eye. Well, in fact it must emerge at lots of different angles, because the arriving light is hitting every possible position on half of the raindrop; but you only see the light that happens to be headed towards you.
One raindrop won't give much of an effect, of course. What we hope is going to happen is that large numbers of raindrops will reinforce one another's effect. In fact, we don't need to hope. We know that the reinforcement is happening, because otherwise we wouldn't see a rainbow.
So far, so good. Unfortunately, that explanation doesn't say why we get the special answer of 42 degrees for the primary rainbow, and 50 degrees for the secondary one. That's what prompted me to do the calculations that are contained in the following sections. I couldn't truly believe in the existence of a rainbow until I had convinced myself that the numbers came out right.
In what follows I'm going to assume that raindrops are spherical. That's not precisely correct. The shape gets distorted by the combined effects of gravity and atmospheric drag. Luckily for the theory, the attraction of water molecules to one another – the effect normally known as “surface tension” – is strong enough to keep the drop's shape pretty close to spherical, especially for the smaller droplets.
When light passes from air to water, it undergoes a change of direction as given by the well-known Snell's law:
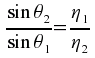
For an air/water interface, the refractive indices are η1 =1.0003 and η2 =1.333.
Now, when the water is a spherical drop, and is reflected once internally, symmetry considerations show us that the exit angle will be the same as the entry angle θ1, as shown in the following diagram. (The light ray will always be in a plane passing through the centre of the sphere. The reason for that is left as an exercise for the reader.) The diagram also shows that part of the light passes right through the drop – because the geometry of the situation, together with Snell's law, implies that θ1 can never be large enough for total internal reflection – but that is of no interest to us, because the light that passes through is in a direction away from the observer.
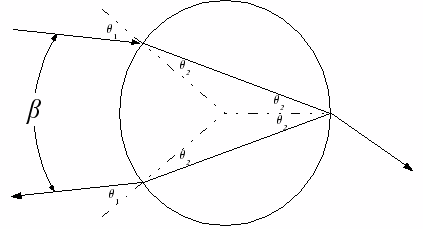
There is a net change of angle β between the incident and returning ray. With the help of a little trigonometry, it turns out that
![]()
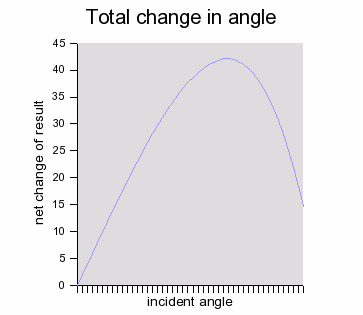
We can plot this as shown on the graph. As θ1 varies from 0 to 90 degrees, β rises to a peak value of about 42 degrees, and then falls again.
Now, look at this from the point of view of an observer whose back is to the sun. That 42 degrees defines a circular arc within which the sky will look brighter, because of the light bouncing back. (There will also be some light that is directly reflected from the outside of the raindrops, without any refraction; but that will have no noticeable effect because it is more uniformly distributed around the sky.) Outside that 42 degree arc the sky looks a little darker, because in that region the light refracted through the raindrops cannot reach the observer.
Because the graph has an inflection point, there is also a focusing effect: at the maximum, a small range of resulting angles corresponds to a larger range of θ1. Thus, the light is brightest at the boundary.
So far, we have ignored the fact that the refractive indices vary slightly with the light frequency. Within the bright region of the sky this will have no visible effect, because light from neighbouring raindrops will combine to give a net white light. At the 42 degree boundary, however, the cancellation doesn't quite happen, because the boundary angle is a little more for red light and a little less for blue light. Because the boundaries don't coincide, we get a rainbow. The main reason why we see the colours so clearly is the focusing effect noted in an earlier paragraph.
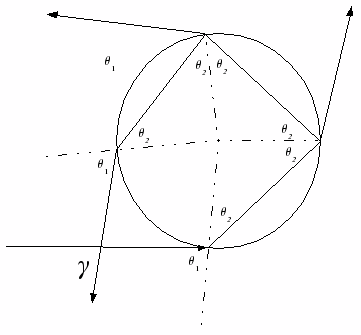
It is fainter because light energy is lost (by
passing through the back of the drop) on both reflections. In this
case the angle change is given by the formula
![]()
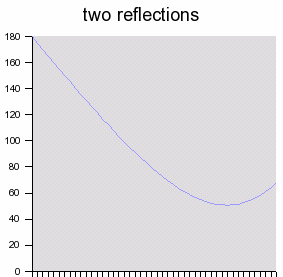
The graph of this angle as a function of θ1 again has an inflection point – and therefore also a focusing effect – this time at about 50 degrees. Note, however, that the inflection point is a minimum of the function, instead of a maximum, and this has two consequences. First, it means that the secondary rainbow is inside out, with red on the inside and violet on the outside. Second, it means that the sky appears marginally brighter outside the secondary rainbow. That means that the dark part of the sky lies in the 8-degree region between the two rainbows.
In principle we can get a tertiary rainbow – that is, one that results from light reflecting three times inside the raindrop. I won't bother drawing the diagram this time. You can try it for yourself if you like. Anyway, the formula for the angle change is easily found to be
![]()
If you plot this as a function of θ1 you will find that it has a maximum, so there should be a third rainbow. The catch is that the maximum occurs where δ is about 222 degrees. That puts it on the other side of the sky from the first two rainbows. To see it, you would have to face the sun. It would be displaced from the sun by about 42 degrees.
In practice, you can't see it during the day. Light intensity is lost at each of the three reflections, so that the final result is very faint. It has no chance of competing with the direct sunlight.
You can sometimes see a ring around the moon when the conditions are right. It appears white rather than coloured, but that's only because humans don't have good colour vision at night. This ring is not, however, the tertiary rainbow. It is actually caused by refraction through ice crystals. Ice crystals are hexagonal, not spherical, so the effects are different from those discussed in this article. Perhaps the tertiary rainbow is also present, but if so it is too faint to be noticed.
Remarkably, higher-order rainbows can also exist. I haven't bothered to take the analysis beyond the seven-reflection case, but in all cases up to that point the change of angle shows a minimum or maximum. Of course, the amount of light that gets through in these cases is so small that it seems unlikely that anyone could see these higher-order rainbows against the general background of the sky.
This article
by Peter
Moylan
Other
articles